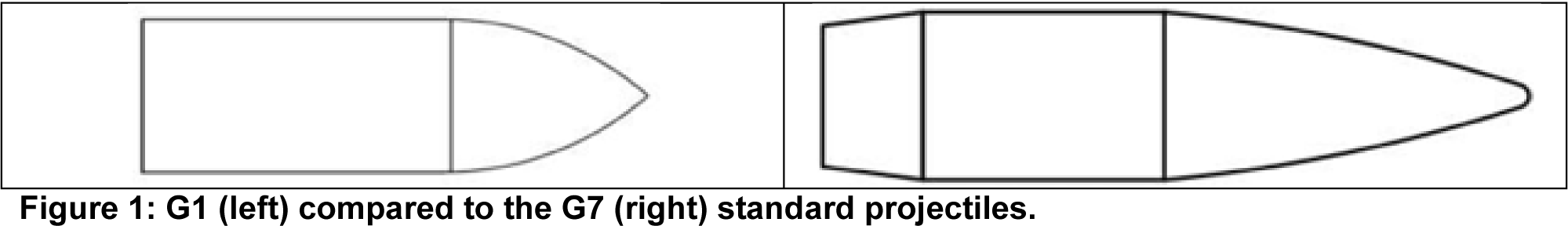
Background
In 2009, Berger Bullets introduced G7 BC’s for boat tail bullets. For those who are unfamiliar with G7 BCs, it’s simply a Ballistic Coefficient referenced to the G7 standard projectile instead of the G1 standard projectile. The G7 standard is a better match for modern long range bullets, so the G7 BC will be more constant over a wide range of velocities compared to a G1 BC. Please refer to article: https://bergerbullets.com/a-better-ballistic-coefficient/ for the complete explanation of the benefits of using G7 BC’s.
Since 2009, many shooters have realized the benefits of G7 BC’s thru more accurate trajectory predictions. The objective of this article is to highlight another empowering analysis feature of the G7 paradigm: form factors.
How Sectional Density and Form Factor comprise BC
In words, the Ballistic Coefficient of a bullet is it’s sectional density divided by its form factor. Sectional density is easy to calculate because it simply depends on the bullets caliber and weight. For example, the sectional density of a 175 grain .308 caliber bullet is: 175/7000/(.308^2) = 0.264 (the bullet weight is divided by 7000 to convert from grains to pounds). Anyone with a pocket calculator can easily figure out the sectional density of any bullet given it’s caliber and weight.
Form factor is the tricky part because it requires a measurement of the bullets drag, which is related to the bullet’s profile. In particular, the form factor is the bullets drag divided by the drag of a standard bullet. When working with G7 BC’s, you divide the drag of a particular bullet by the drag of the G7 standard projectile.
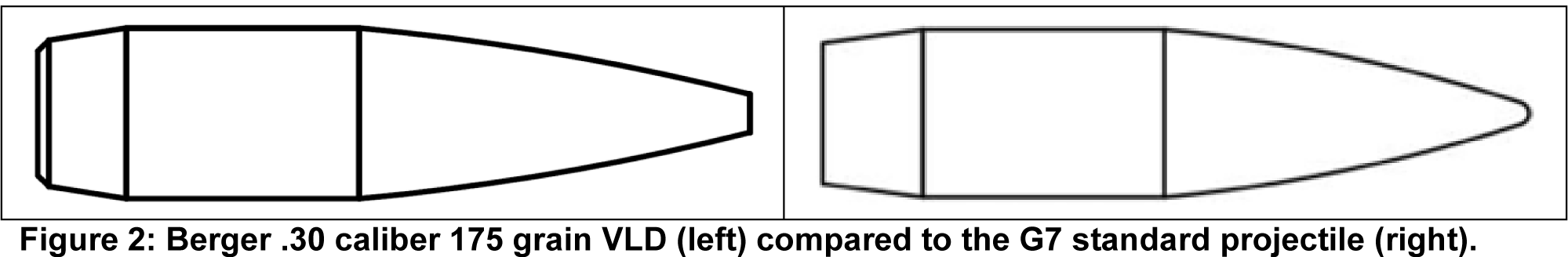
As an example, consider the .30 caliber 175 grain VLD Target pictured below next to the G7 standard projectile.
Looking at the two bullet profiles, not considering the caliber or weight, how would you expect the drag of the Berger VLD (on the left) to compare with the drag of the G7 standard (on the right)? Well, the VLD has a shorter boat tail, and a blunter nose than the G7 standard projectile, so it ought to have more drag. In fact, the measured G7 form factor of this VLD is 1.006. That means the drag of the VLD is 1.006 times the drag of the G7 standard (In other words, 0.6% more drag).
To calculate the G7 BC of this bullet, simply divide it’s sectional density, .264, by the form factor of 1.006: .264/1.006 = .262.
I apologize for the math involved in this explanation, but please stick with me. I promise it will be worth it!
Getting a feel for form factor
The following table will broaden the application of form factor to several other bullets that have different profiles with different amounts of drag.
The first bullet that appears at the top of the chart has a very short boat tail, and a short ogive with a wide blunt tip. This bullet has a form factor 1.286, or 28.6% more drag than the G7 standard shown in the middle of the chart.
The next bullet down has a longer nose (lowers drag), with a smaller diameter tip (also lowers drag), but still has a relatively short boat tail. This bullet has a form factor of 1.036, or 3.6% more drag than the G7 standard.
The next bullet down has a long nose and boat tail. Note that the length of the bearing surface is unimportant to the bullets drag and form factor. Only the nose length, nose profile, meplat diameter, boat tail angle and boat tail length dictate what the drag and form factor of the bullet will be. This bullet has a form factor of 1.006, which is only 0.6% more drag than the G7 standard; essentially identical. Since this bullet has a form factor so close to 1, it will have a G7 BC that’s very close to its SD (because BC = SD/form factor).
Next down is the G7 standard. The weight and caliber of this bullet is unimportant in this analysis of form factors.
Next down is a bullet with a long nose and BT with a G7 form factor of 0.993, again very close to 1.000, but just a little bit less drag than the G7 standard (0.7% less drag to be exact).
Next is a bullet with a very long secant nose, small meplat and long boat tail. This bullet has a G7 form factor of 0.933, which is really very low drag. The last bullet has a nose and boat tail very similar to the bullet just above it, but has a form factor of 0.923. That’s 7.7% less drag than the G7 standard and is considered very good.
Applying the knowledge of form factors, or: “What’s it all mean?”
For those who’ve stayed with the conversation this far, thank you. Your long attention span is about to be rewarded!
We’ve discussed what the G7 form factor is: a factor that relates the drag of any bullet to the drag of the G7 standard projectile. So why is it so important to have an awareness and understanding of form factor? Isn’t this what BC’s are for; to be able to make comparisons between bullets using a single number? It’s true that BC is a useful measure of merit for ballistic performance, but there’s a problem with using BC’s alone to assess ballistic performance. The problem with BC’s is that they combine the effects of mass and drag into one number. So if a bullet has a high BC, you don’t know if it’s a medium weight bullet with very low drag, or a heavy bullet with high drag. The reason this is important is because if a bullet has a high BC just because it’s heavy, it will suffer from having a depressed muzzle velocity, and performance will not be as good as the high BC implies.
To illustrate the importance of this, consider two .30 caliber bullets. One is 175 grains with a very low drag profile (low form factor). The other is a 190 grain bullet that is a higher drag profile. Both bullets have the same BC. What bullet would you rather shoot, and why?
In this example, the obvious choice is the 175 grain option because you will get higher muzzle velocity and still have the same BC.
Bullets will achieve different muzzle velocities depending on their weight; with lighter bullets achieving higher muzzle velocities than heavier bullets. This makes it difficult to assess ballistic performance for bullets of different weights, just based on their BC alone.
However, form factor is a more universal indicator of a bullets efficiency and performance potential. The form factor of a bullet is essentially a measure of how efficiently a bullet flies, regardless of the bullet’s weight.
Looking over the line of Berger Bullets, examples of low form factor bullets are:
• The 6mm 105 grain Hybrid Target with a form factor of .925
• The 6.5 mm 130 grain VLD Hunting with a form factor of .925
• The 7mm 180 grain Hybrid Target with a form factor of .915
• The .338 300 grain Hybrid OTM Tactical with a form factor of .891
If you’ve ever heard someone make a comment to the effect of: “it’s a high BC bullet for its caliber and weight“, what they’re essentially saying is that bullet has low drag, and a good (low) form factor. These are the bullets you want to identify because they will give the best ballistic performance, regardless of what weight or caliber the bullet is, and what MV you can achieve with it.
Bullet mass basically trades retained velocity for muzzle velocity, which is essentially a wash in terms of ballistic performance. However, low drag makes a bullet of any weight more efficient at any velocity.
If you’re wondering how to figure out the G7 form factor of various bullets, it’s quite simple. Just divide the sectional density by the G7 BC. For example, consider the .30 caliber 175 grain bullet used earlier in this example. It’s sectional density is .264, and the G7 BC is .262. So the G7 form factor is .264/.262 = 1.006. In this way, you can calculate the form factor for any bullet you have a G7 BC for, and assess that bullets efficiency in terms of form factor.
Currently, Berger is one of the few bullet companies that provide G7 BC’s for their bullets. However, I’ve published a book that has experimentally measured BC’s for over 235 bullets from many brands, including their G7 form factors. If you want to save yourself some time doing calculations, you can pick up the book to see a tabulated list of all measured bullets with their G7 form factors.
Following is a table that lists the G7 form factors for all boat tail Berger Bullets (the G7 standard applies best to boat tail bullets, whereas the G1 standard applies for flat base).
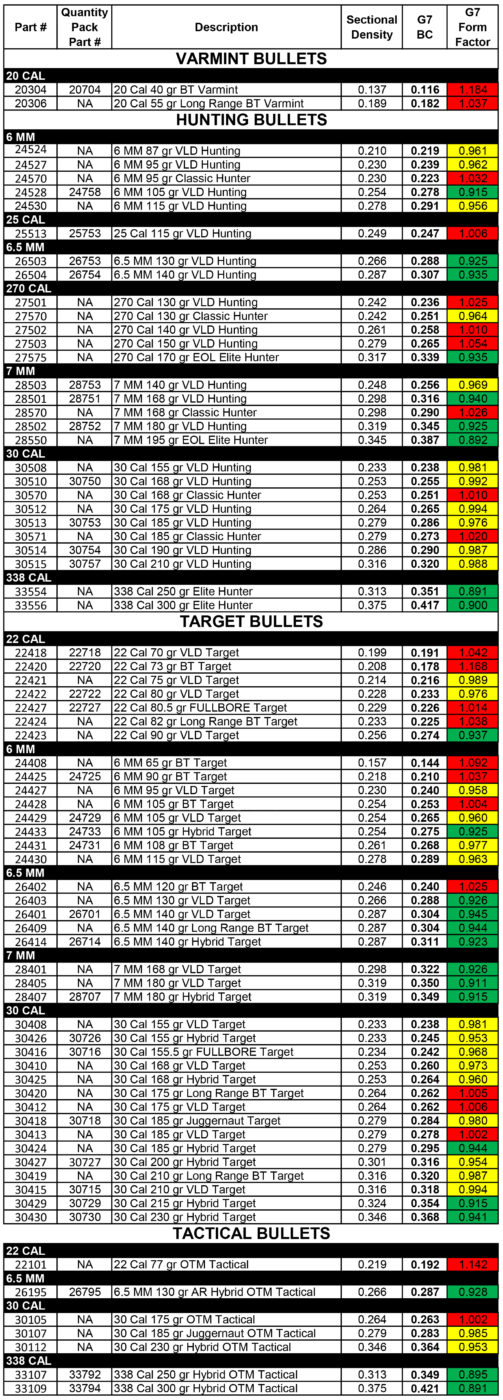
Form factors in the QRS are based on un-rounded calculations and can vary by 0.001 compared to calculations done using rounded values of SD and BC.
This table shows the basic metrics for all Berger Bullets that have G7 BC’s (bullets with boat tails).
You’ll notice that the sectional densities and BC’s vary a great deal for all these bullets because of the different weights and calibers. It’s impossible to know from the BC alone if a bullet is a good bullet for a particular caliber and weight.
However, the form factor is unrelated to the caliber and weight, so it clearly indicates the merit of the bullets profile, as it relates to low drag and ballistic performance.
The form factors are color coded according to the G7 form factor.

Bullets with form factors in the green category are extremely low drag and quite rare among the many various brands of long range bullets. You can see that Berger’s line has the highest concentration of ‘green’ in the 6.5 and 7mm calibers. That is one reason why these calibers are so successful at long range, because they have bullets with relatively high BC’s for their caliber and weight; which is a direct effect of the low form factor (low drag).
Conclusion
• The analysis of form factors can be very useful when considering a bullets long range performance potential.
• Going by BC alone can be deceptive since BC includes the weight and caliber of the bullet.
• Form factor indicates how much drag the bullet has, which is a very important consideration for all bullets of all calibers.
• Unlike BC, knowledge of form factors is universal among all calibers and weights of bullets. A G7 form factor of 0.920 is excellent for any bullet, be it .22 cal, 6mm, or .338 caliber.
• Form factor is not dependant on bullet weight or caliber.
Next time you’re considering the performance potential of a bullet for long range shooting, be sure to ask yourself how the form factor compares to other bullets in its class. Look for more low form factor/high BC bullets to be made available from Berger in the coming months and years.
Bryan Litz
Chief Ballistician
Berger Bullets